There are a number of ways to approach the sinusoidal/periodic function. We were taught to use either GRAPHMATICA or a graphing calculator.
The periodic function equation is y=Asin(Bx+C)+D and that is what you will input into the software of your choice.
A=Amplitude
B=Period (formula for period P=2(pi)/B)
C=Phase shift
D=Verticle shift
Here is a site that demonstrates those values (very usefefull to play around with it)-http://www.ronblond.com/M12/sc.APPLET/index.html
If you are given the sinusoidal function(periodic function) and want to graph it using a calculator
1. press[mode] and make sure that it is on radian NOT degrees
2. press [y=] and input the formula Asin(Bx+C)+D (substitue numbers)
3. press [graph] and behold the squiggly lines
-you may want to adjust the windows setting to get a better picture
If the information is given in tabular data then you will have to use something called SinReg
1. press [stat] and put the data into a list
2. press [stat]-> calc then go down to something called SinReg
3. back at the home screen put in SinReg,L1,L2,Y1
4. turn on the stat plot ([2nd y=]) and press graph
And that my friends is periodic functions in a nut shell.
Grade 12 students in Manitoba learning Applied Math... They're in two different towns, and happen to be connected by Highway 10...They'll be learning with and from each other. Ryan Maksymchuk and Cam Bennet are teachers in Swan River and Dauphin, Manitoba, respectively. This is a bright idea that may encourage other teachers and students to consider collaborating with other learners in other places...It might work. It might not...Watch and see...
Tuesday, June 23, 2009
Tuesday, June 2, 2009
Sequences and Series Review
Ok, this is a little late. I would use slideshare for this but, it doesn't want to work nicely. I'll just give you a download link while I fix slideshare.
Sequences and Series PPT
(Sept 10/09)
Update: The PPT link actually works out pretty well and if I were to change the Show to be nice with Slideshare I would have to change quite a bit. I'm just going to leave it the way it is.
Sequences and Series PPT
(Sept 10/09)
Update: The PPT link actually works out pretty well and if I were to change the Show to be nice with Slideshare I would have to change quite a bit. I'm just going to leave it the way it is.
Monday, June 1, 2009
OK guys were going to work with Vectors. Now it's pretty easy... A Vector is anything with a direction and a magnitude. First thing to do is draw a rough looking diagram just to show you more less what is going to look like and to make sure that your on the right track.
Some key words and phrases are:
If you know your directions your going to get this...
These are the few different directions that your going to end up getting....
Always start with the first direction you are given and go clockwise or counter clockwise in the amount of degrease you are given.
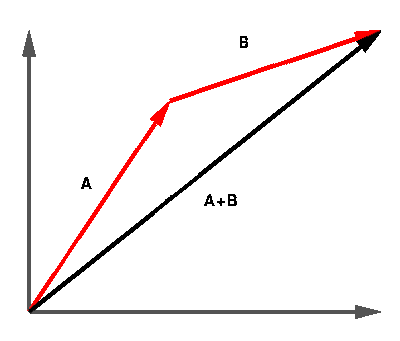
As you see the first direction it is done as i said but the second direction is a bit more tricky. When you draw you first line just draw it till the distance you need to go. For example it may say 5km when of coarse you couldn't draw it. Make a scale that makes since to the amount of space you have to draw it. 1km may be one centimeter. When you draw your second line you must imagine or draw a norther grid as the end of line and draw the second line just like the first.
If the question asks you what is the distance, "the way the crow flys" it's as simple as measuring the distance back to the staring point. If you have drawn it to scale and wrote down the right distances just use a ruler for the distance.
Some key words and phrases are:
One of the things you going to run into is a ''Bearing" a bearing will be given to you in a number, that number is the amount of degrees it is clockwise from north. For example a bearing of 90 would mean that it would straight east.
If you know your directions your going to get this...
These are the few different directions that your going to end up getting....
North of East
East of North
East of South
South of East
South of West
West of South
West of North
North of West
East of North
East of South
South of East
South of West
West of South
West of North
North of West
For example if you have "North of East" you would look at the north spot and travel East. Now to throw something else in there is going to be a number like "North 45 East"
Always start with the first direction you are given and go clockwise or counter clockwise in the amount of degrease you are given.
When your writing your question down you have to be as accurate as possible because you will be given two or more directions and have to measure the distance back...

This is Vector addition
As you see the first direction it is done as i said but the second direction is a bit more tricky. When you draw you first line just draw it till the distance you need to go. For example it may say 5km when of coarse you couldn't draw it. Make a scale that makes since to the amount of space you have to draw it. 1km may be one centimeter. When you draw your second line you must imagine or draw a norther grid as the end of line and draw the second line just like the first.
If the question asks you what is the distance, "the way the crow flys" it's as simple as measuring the distance back to the staring point. If you have drawn it to scale and wrote down the right distances just use a ruler for the distance.
Good luck...
Stats unit review, (confidence intervals)
This is a piece of the STATS unit review.
Confidence intervals.
A confidence interval is… “Predicting the likelihood that the mean of a sample occurs within a certain interval, a certain percent of the time”.
90%...95%...99%... sizes of confidence intervals considered “worthwhile” for statistical prediction purpose.
Here is an example question:
A research study on fruit flies found that an average of 50% of them stay alive after 32 hours.
a) Determine the 95% confidence interval for the percentage of a sample of 1000 fruit flies still alive after 32 hours. Show your work.
Here is what you do.
In your calculator, press the buttons… STAT then TESTS. Go to Prop z-int and it brings up this screen.
X:
N:
C-Level:
Calculate
X is your mean, N is your total number, so in this example your X would be 500, and your N would be 1000. Your C-level is 95. Press calculate and you should get this screen.
1-PropZInt
(.046901,.053099)
P=.5
N=1000
Your percentage interval is (.046901,.053099)
After every question you need to explain the meaning of the confidence interval. So in this example you would write….
If a similar experience/survey/poll were conducted this interval (46.9, 53.1) means that we are 95% confident that 32 hours later out of the 1000 fruit flies between 46,9 and 53.1 percent of them will remain alive.
I hope you are confident with this part of the unit, and if you have any questions please place them in the comments section and I will do my best to answer them.
Confidence intervals.
A confidence interval is… “Predicting the likelihood that the mean of a sample occurs within a certain interval, a certain percent of the time”.
90%...95%...99%... sizes of confidence intervals considered “worthwhile” for statistical prediction purpose.
Here is an example question:
A research study on fruit flies found that an average of 50% of them stay alive after 32 hours.
a) Determine the 95% confidence interval for the percentage of a sample of 1000 fruit flies still alive after 32 hours. Show your work.
Here is what you do.
In your calculator, press the buttons… STAT then TESTS. Go to Prop z-int and it brings up this screen.
X:
N:
C-Level:
Calculate
X is your mean, N is your total number, so in this example your X would be 500, and your N would be 1000. Your C-level is 95. Press calculate and you should get this screen.
1-PropZInt
(.046901,.053099)
P=.5
N=1000
Your percentage interval is (.046901,.053099)
After every question you need to explain the meaning of the confidence interval. So in this example you would write….
If a similar experience/survey/poll were conducted this interval (46.9, 53.1) means that we are 95% confident that 32 hours later out of the 1000 fruit flies between 46,9 and 53.1 percent of them will remain alive.
I hope you are confident with this part of the unit, and if you have any questions please place them in the comments section and I will do my best to answer them.
Subscribe to:
Comments (Atom)



